On this page, we present our most recent news, ordered by date. For browsing our catalogue of math sculptures in a more structured way, either use the menu bar or a search field.
January 2025
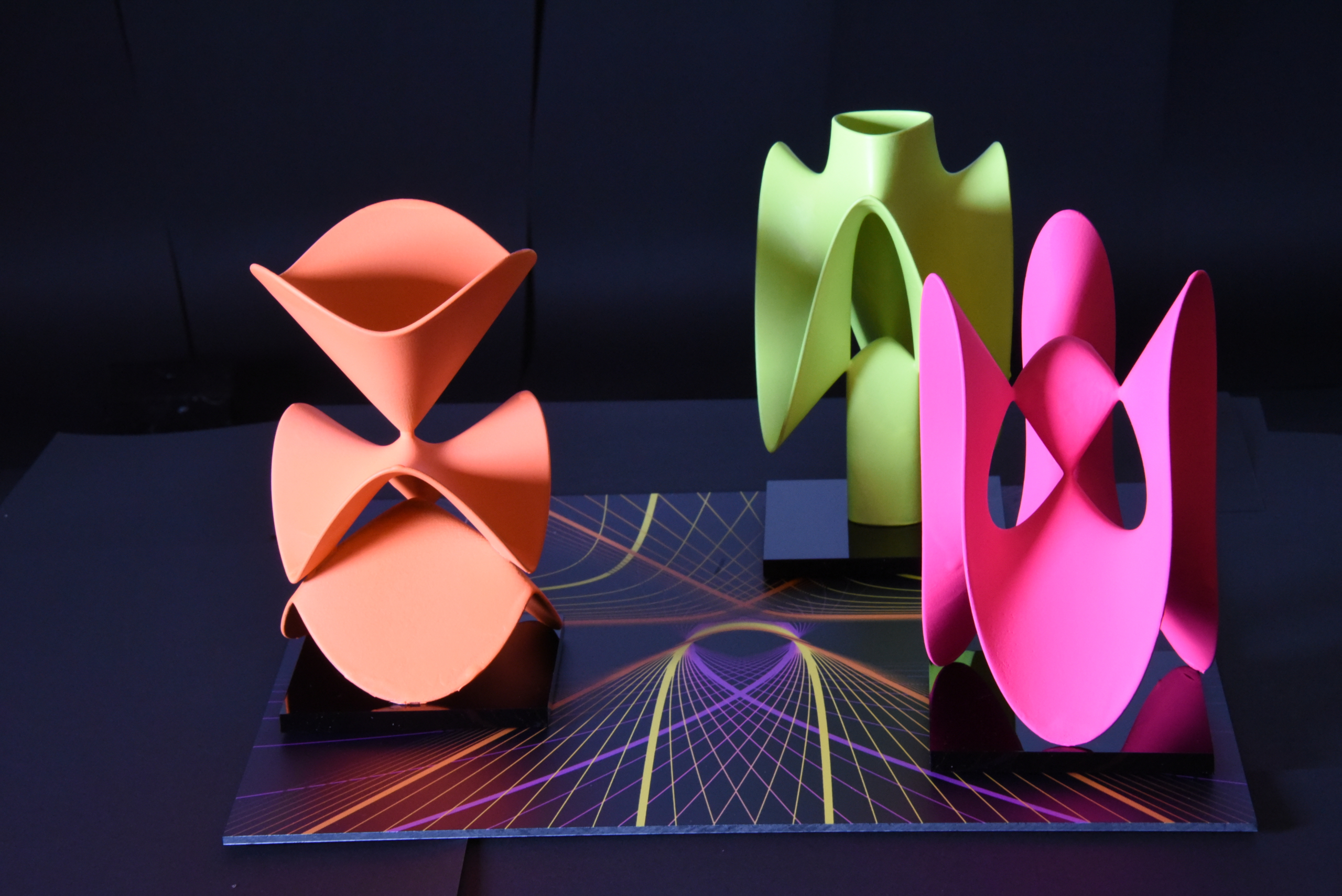
Three views of a projective cubic surface
In January 2025, Oliver Labs created a corresponding group object composed of three 3d printed sculptures standing on a planar visualization of this family of curves for an exhibition at the Planetarium, Hamburg, Germany. [...]
December 2018
Equations for lines and circles
This post explains briefly how lines and circles may be described by equations. A particular focus are implicit equations which will play an important role in later posts in the category "equations and shapes" on math-sculpture.com.
November 2018
Equations and Shapes
This post explains briefly how lines and circles may be described by equations. A particular focus are implicit equations which will play an important role in later posts in the category "equations and shapes" on math-sculpture.com.
January 2018
A Quintic with 15 Cusps
The quintic with 15 cusps is a so-called world record surface: To our knowledge, it is not known if there may be a quintic with more than 15 cusps although it is known that a quintic cannot have more than 20 cusps.
November 2017
45 cubics, series II (cylinder cut), 15cm
The complete series of 45 types of cubic surfaces with only finitely many singularities and lines. We provide links to put a complete set of several cubic surface models into your shopping cart.
July 2017
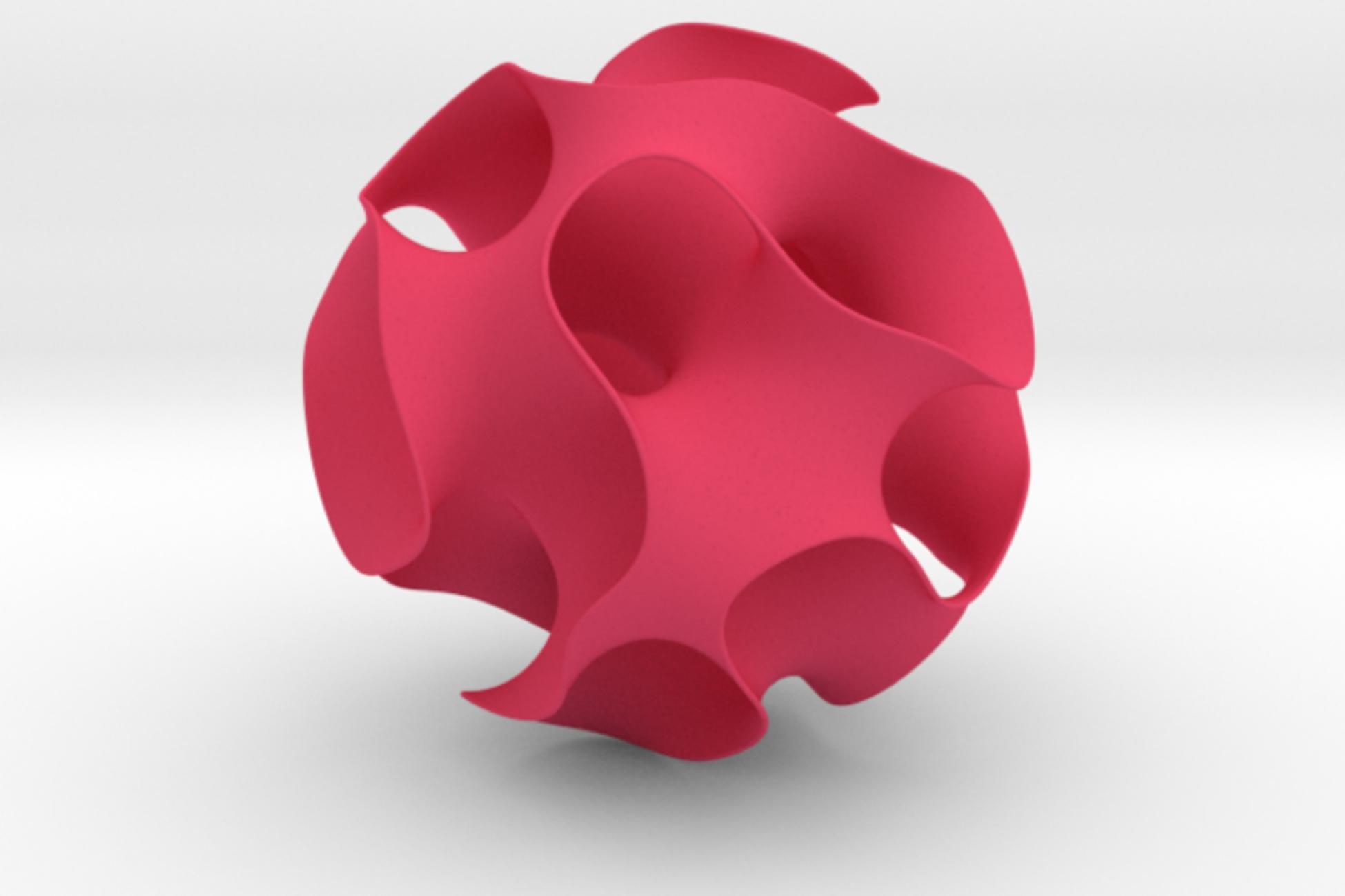
A gyroid, round cut
The gyroid is a modern classic. It is a so-called minimal surface. Our object is an approximation of it in terms of sine and cosine. This one shows more tunnels than our other version.
March 2017
Moebius Strip ring
The Moebius Strip is a mathematical classic, and even for rings it has been used for many decades at least. Our version of the Moebius Strip ring, however, is special. Its shape is defined by a mathematical formula!
Moebius Strip pendant
The Moebius Strip is a mathematical classic, and even for pendants it has been used for many decades at least. Our version of the Moebius Strip pendant, however, is special. Its shape is defined by a mathematical formula!
Moebius Strip pendant
The Moebius Strip is a mathematical classic, and even for rings it has been used for many decades at least. Our version of the Moebius Strip pendant, however, is special. Its shape is defined by a mathematical formula!
A math vase of degree 3 without bottom
A math vase of degree 3 without bottom. It has been created by rotating a graph of a polynomial of degree 3 about an axis.
A math vase of degree 3
A math vase of degree 3. It has been creating by rotating a graph of a polynomial of degree 3 about an axis.
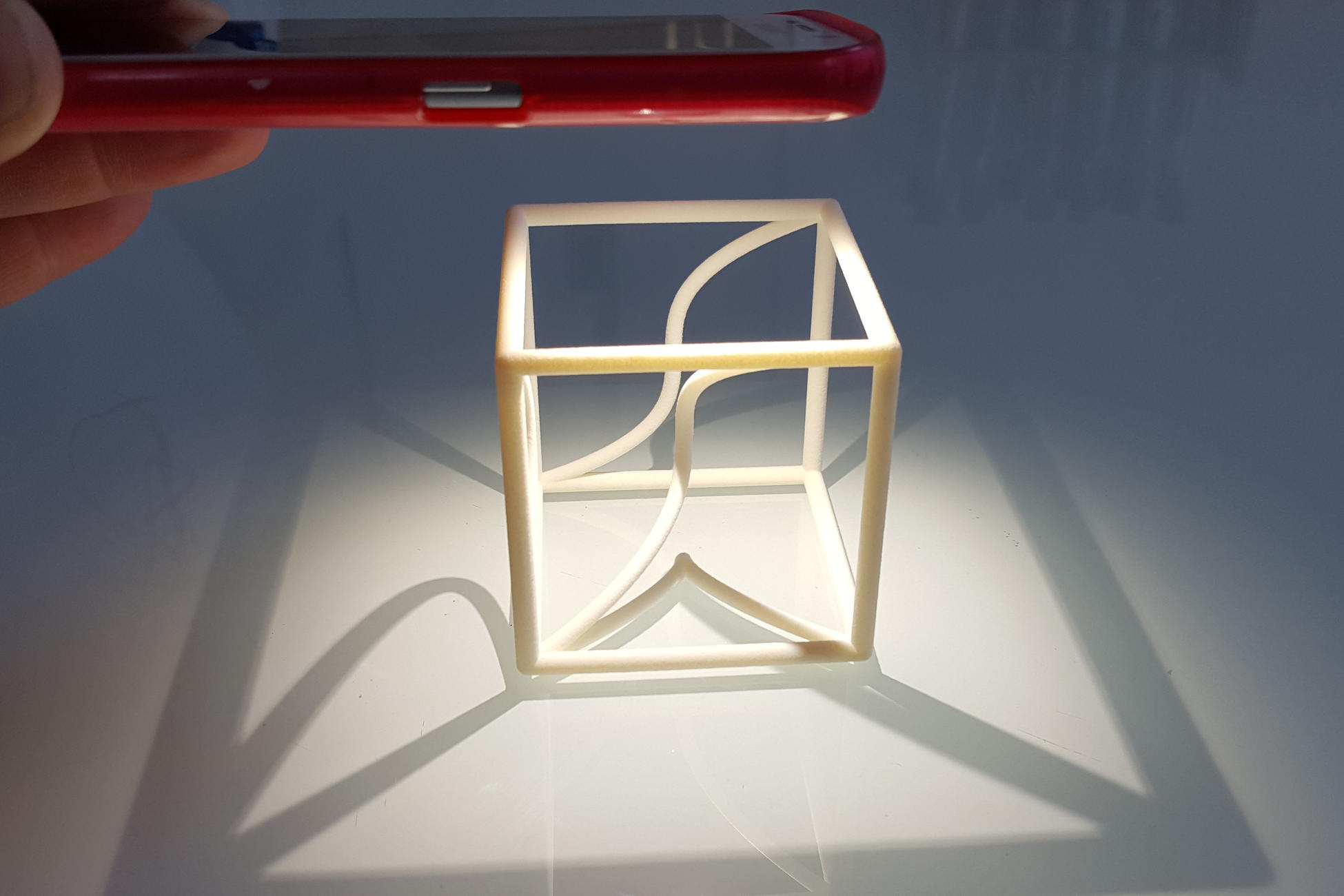
Space curve in a cube with projections (1b)
This space curve in a cube with projections (1b) is a math classic. Use a torch to compare the projections of the space curve (in the center) on a plane with the 3d-printed ones.
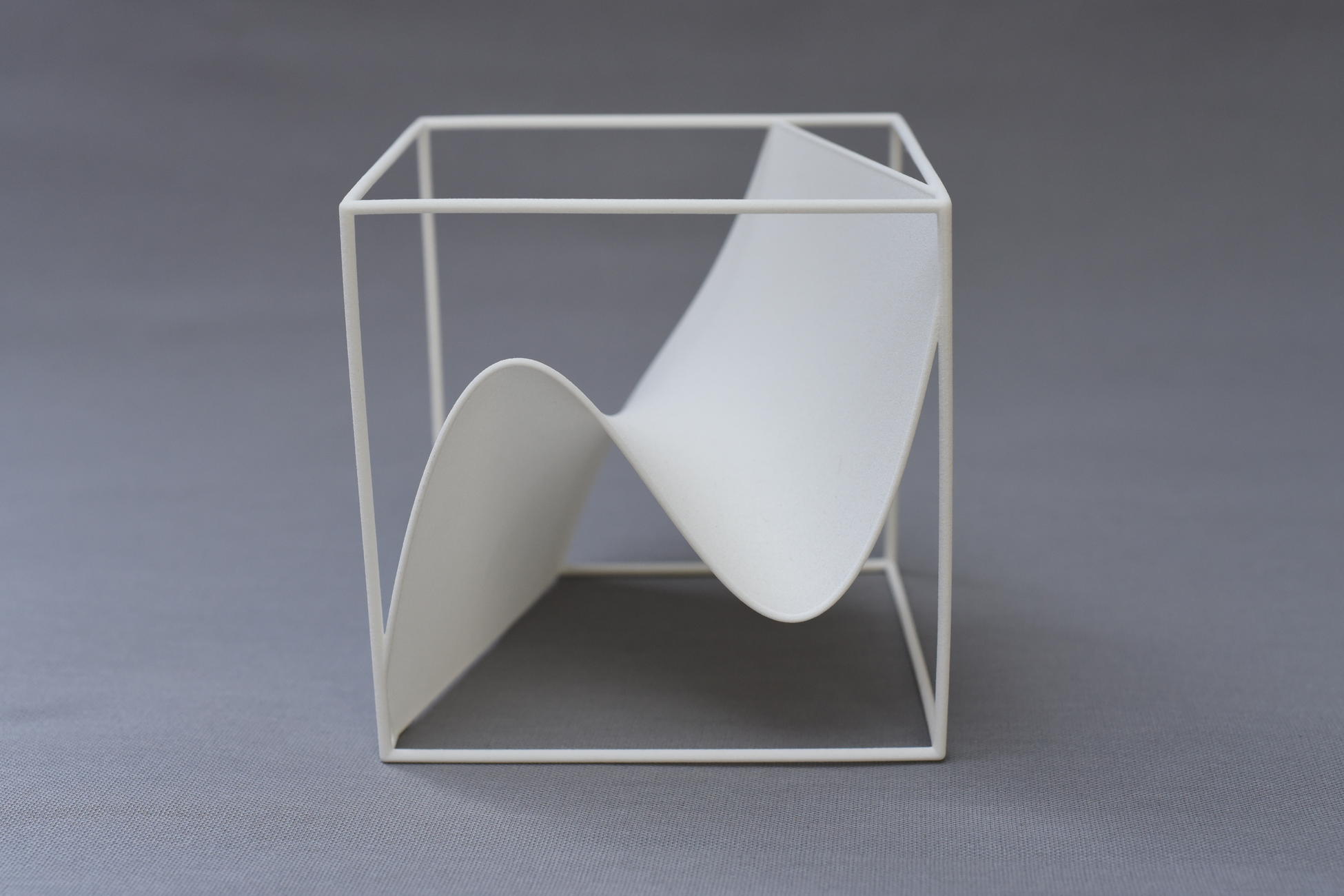
Space curve in a cube without projections (1a)
This space curve in a cube is a math classic. To view its projections on a plane, just take a torch (or your cell phone lamp).
A trefoil knot pendant
A trefoil knot is the simplest non-trivial mathematical knot. It has been known for thousands of years.
Cubic surface KM 42 pendant
Cubic surfaces are a math model classic from the 19th century. We provide one of our favourite examples (cubic surface KM 42) in the form of a pendant.
Speed Curve pendant
At first sight, our speed curve pendant might seem to consist of some arbitrary wire. But this is not true at all. Almost every detail is defined by mathematical formulas.
The Clebsch diagonal surface: 27 lines only
The sculpture we present here is a 3D-printed modern object consisting of the 27 lines only, and a thin part of the surface as a border.
February 2017
A gyroid, round cut
The gyroid is a modern classic. It is a so-called minimal surface. Our object is an approximation of it in terms of sine and cosine.
A 3d graph of a cubic function
This visualizes a 1-parameter family of cubic functions or a 3d graph of a function in one variable in a 3d-coordinate system.
Boy and Möbius in the family room
The Moebius strip is a simple, but fascinating math object, with just one side and one connected boundary curve. Werner Boy's surface contains such strips!
45 cubics, series II (cylinder cut), 12cm
The complete series of 45 types of cubic surfaces with only finitely many singularities and lines. We provide links to put a complete set of several cubic surface models into your shopping cart.
45 cubics, series I (ball cut)
The complete series of 45 types of cubic surfaces with only finitely many singularities and lines. We provide links to put a complete set of several cubic surface models into your shopping cart.
Barth’s sextic
The Barth Sextic is probably the most famous example of the sometimes so-called world record surfaces. This post shows a smoothed variant of it.
Sylvester’s amphigenous surface
In the 19th century Sylvester described a surface for studying the number of real roots of a polynomial. Henrici later constructed a model of this. This is a modern version.
The Clebsch diagonal surface with colored lines
This version of Clebsch's famous diagonal surface model features colored lines. One intersects the surfaces in a line and a hyperbola, the another one in three lines.