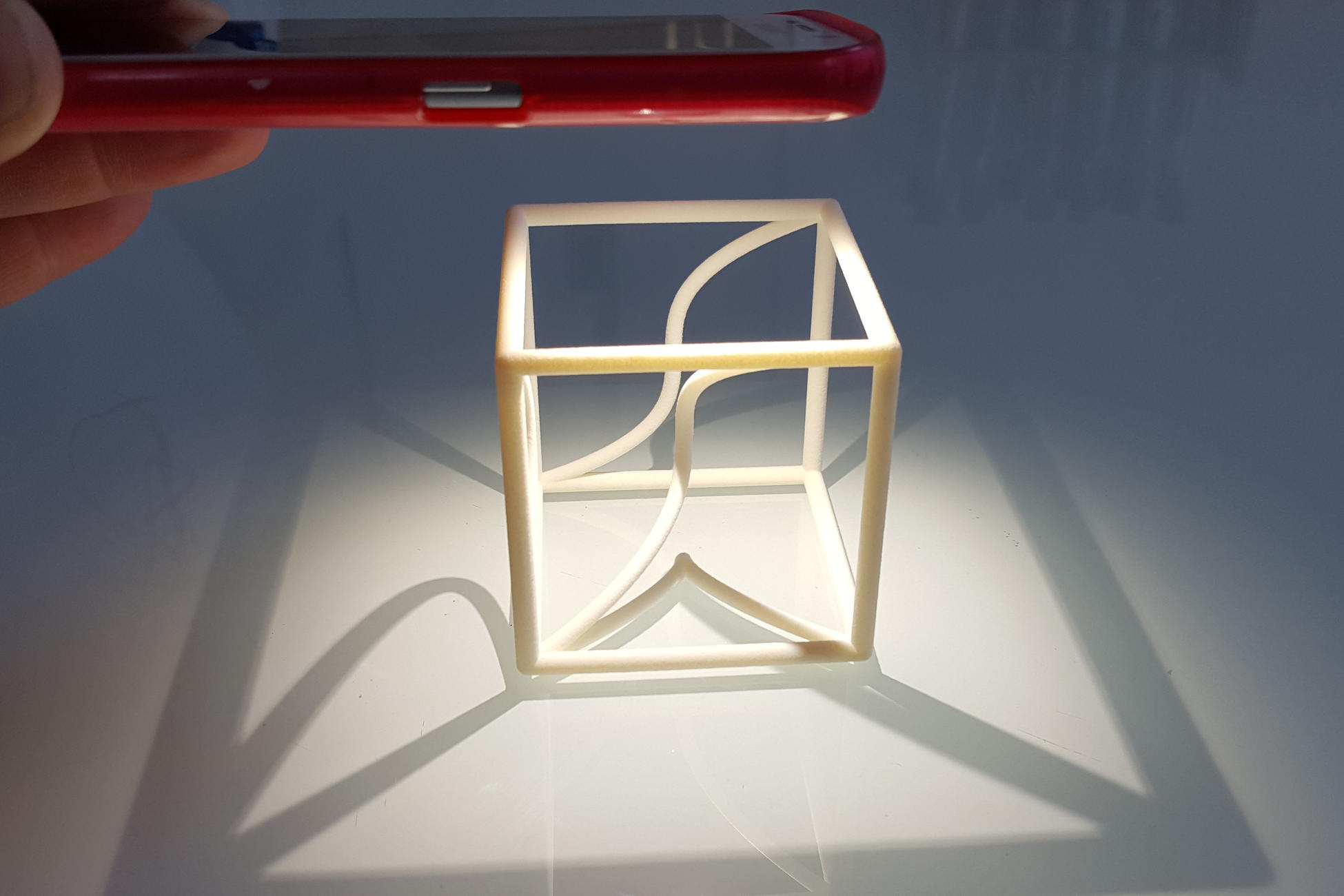
This space curve in a cube with projections (1b) model is a math classic. To view the projections of the space curve (in the center) on a plane, just take a torch (or your cell phone lamp).
Its projections to the sides of the cube are particularily interesting, one is a standard parabola, another one some graph of a polynomial of degree three, and the third one is a curve with a so-called cusp. These plane curves are shown on three of the sides of the cube, so that you may compare them with the shadow created by your torch.
In mathematics, the particular curve used for this model, is called rational normal curve, with parametrization $(t,t^2,t^3)$.

The construction of such mathematical models of curves with projection goes back at least to the year 1879 when Christian Wiener created  eight models illustrating mathematical space curves. These had an interesting shape at the origin which projects interestingly onto three coordinate planes.

To purchase our 3d-printed modern version of this model use the “buy now” link provided below. You will be sent to our online shop at shapeways where you will be able to choose a size and color. Your object will then be 3d-printed just for you and sent to you in really short time where ever you are in the world.

We also created a math model which is identical apart from the fact that the three mentioned projections are not shown in the model. This post and others related to algebraic space curves models are shown below: