A photo from our series “Math sculptures in context”. To purchase the models used in the photo and others, follow the links to our producer “shapeways” provided below.
One of the best known mathematical models among mathematicians is the so-called Boy surface because a more than human-sized metal version is standing in front of the mathematical research institute at Oberwolfach, Germany.
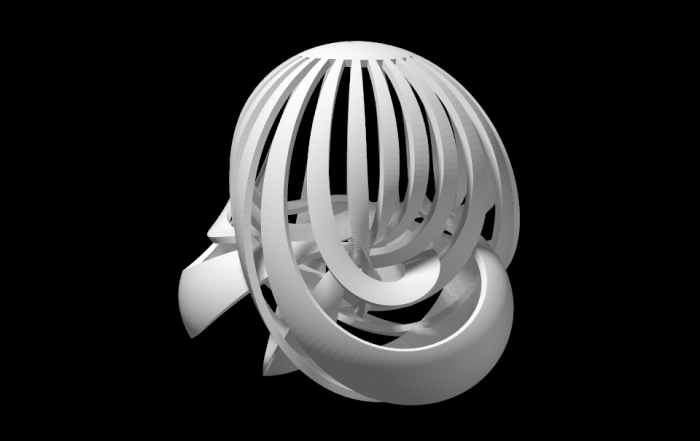
The surface is named after Werner Boy who wrote his dissertation in 1902. David Hilbert had given him the task to prove that there is no so-called immersion of the real projective plane in three-space. But surprisingly, Boy constructed such an immersion. To honor this achievement, all such surfaces are now called Boy surfaces. The Boy surface is non-orientable as the well-known Möbius strip; in fact, it contains a Möbius strip. You obtain such a strip by taking an ordinary strip of paper, turn one of the ends by 180 degrees and then glue the two ends of the strip. The resulting surface does not have a clearly defined inner or outer side, but just a single one.
Our photo shows a white 3D-printed modern version of the Boy surface which is based on a much more recent parametrization found by Robert Bryant. The same object is engraved inside a piece of glass at the right. The jewelry in the picture are different kinds of Möbius strips. The one next to the wall is essentially an ordinary Möbius strip, but its parametrization is chosen carefully such that it can be used perfectly as a ring. The other one on the table is also non-orientable, but its shape can be obtained in a way similar to the Möbius strip, only the strip is turned three times by 180 degrees before glueing; the example from the photo is a beautiful pendant.
All these MO-Labs math objects are sitting in front of Boy’s and Möbius’ portraits. The setting was created in the MO-Labs office, inside a dollhouse built just for taking photos of math sculptures in historical environments. August Ferdinand Möbius’ portrait is taken from wikipedia. Werner Boy’s portrait is more difficult to find; we are not even sure that the presented portrait is correct, but it appears in a recent article on Boy published by his former school where he was a teacher.
MO-Labs sculptures used: The Boy surface
To purchase this version of the Boy surface, just use the “buy now” link provided below. In our MO-Labs shop on “shapeways”, you will be able to choose a material and color before actually putting it into your shopping cart.
MO-Labs sculptures used: The triply turned Möbius strip pendant
To purchase the triply turned Möbius strip pendant, you may use the “buy now” link provided next to the image. You will be sent to our MO-Labs shop on the “shapeways” website, so that you can choose the material and color there, and the let “shapeways” produce the object for you and send it to you in really short time.
Series: Math sculptures in exceptional settings
This February 2017 photo is the second of a monthly series of photos of mathematical sculptures in exceptional settings. We use these and other posts on this site to show, describe, and make available for purchase our several hundred designs of mathematical sculptures.
Some of the models in the photo have their individual posts where we describe some more details:
Moebius Strip ring
The Moebius Strip is a mathematical classic, and even for rings it has been used for many decades at least. Our version of the Moebius Strip ring, however, is special. Its shape is defined by a mathematical formula!
Moebius Strip pendant
The Moebius Strip is a mathematical classic, and even for pendants it has been used for many decades at least. Our version of the Moebius Strip pendant, however, is special. Its shape is defined by a mathematical formula!
Moebius Strip pendant
The Moebius Strip is a mathematical classic, and even for rings it has been used for many decades at least. Our version of the Moebius Strip pendant, however, is special. Its shape is defined by a mathematical formula!
Boy and Möbius in the family room
The Moebius strip is a simple, but fascinating math object, with just one side and one connected boundary curve. Werner Boy's surface contains such strips!
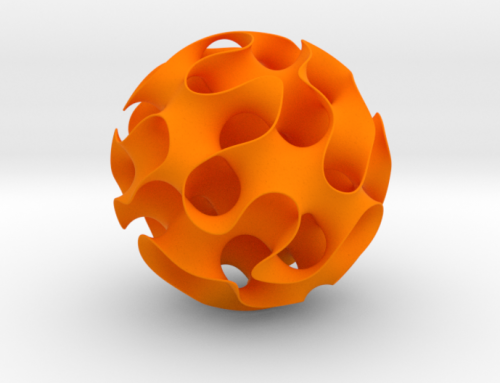
A boy surface, stripes version
This so-called Boy surface represents a fascinating example of a non-orientable surface. The first such surface was constructed by Werner Boy in his dissertation in 1902.










Leave A Comment