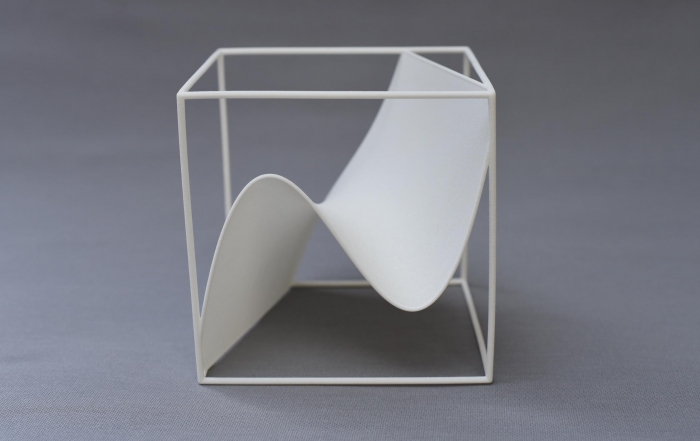
The photo above shows our “45 cubics series II (cylinder cut), 12cm” at Strasbourg university, France, in June 2015. This was the first exposition of our new and more elegant series of cubic surfaces.

Ccubic surfaces have been classified several times in the past. The first reasonable classifications were developed by Ludwig Schläfli in 1858 and 1863.

There is a modern version by Knörrer and Miller from 1987. Their method of classification yields almost the same result. The most interesting part consists of the classification of all irreducible cubic surfaces with only finitely many singularities and lines. They found 45 different types of these. Oliver Labs carefully created equations for each of those types such that all main features of each of the surface can be visualized in a single image or model, called “KM 01”, …, “KM 45”. Here is the article in PDF.

Our series “45 cubics series II (cylinder cut)” presents exactly this classification. As the name of the series suggests, each of the cubic surfaces has been cut by a cylinder in order to get a finite object. This gives the whole series a quite elegant look. This second series has been optimized in several other aspects as well. In particular, we designed all models carefully such that the shapes are as close as possible to the abstract mathematical surface. This means, e.g., that their walls are as thin as possible and the singularities are as close to a point as possible while still keeping the objects producible using 3d printing.

This complete series of all 45 cubics (cylinder cut) with only finitely many singularities and lines was first exhibited at the university of Strasbourg, France, starting from June 2015. Our older cubic surfaces series I (ball cut) was first exhibited at Lisbon from 2012 on.

Links to purchase parts or all models from this series

Below, you may choose your preferred subset of the series of all 45 types of cubic surface models (series II (cylinder cut)). For your convenience, we provide links via which you may put a complete set of several cubic surface models into your shapeways shopping cart:

Buy all 45

Put all 45 models from “45 cubics series II (cylinder cut)” into your cart (these are 45 different cubic surface models of a size of approximately 11.9cm); to check the current contents of your shopping cart, you may open your cart here:

PUT ALL 45 CUBICS SERIES II (CYLINDER CUT)
INTO YOUR SHAPEWAYS CART

Buy a selection of 6 models

If you are just looking for a few of these, here are 6 cubic surface sculptures, carefully selected by Oliver Labs. To put these 6 objects from “45 cubics series II (cylinder cut)” into your shapeways cart, just click the following link; to check the current contents of your shopping cart, you may open your cart here:

PUT 6 SELECTED CUBIC SURFACE MODELS FROM SERIES II (CYLINDER CUT)
INTO YOUR SHAPEWAYS CART: 01, 6, 11, 27, 43, 44

These are the 7 models included in this set:

  • KM 01: a smooth cubic with 27 straight lines. This is NOT the Clebsch diagonal cubic, but a slightly more general cubic, so that that 27 lines have a more general intersection structure.
  • KM 06: a cubic with 1 conical singularity (A1) and 21 straight lines. Comparing this model with number 01, one can see how the singularity forces 12 lines to coincide in pairs.
  • KM 11: a cubic with 2 conical singularities (A1).
  • KM 27: a cubic with 4 singularirities and 9 straight lines. We call this cubic “Cayley/Klein cubic” because it was Arthur Cayley who first studied this cubic in a publication, and it was Felix Klein who presented the first model of it and who based his deformation theory of cubic surfaces on this surface.
  • KM 43: a cubic with 3 cuspidal singularities and 3 straight lines. We call this surface “Salmon’s cubic” because George Salmon was the first to realize that 3 is the maximum possible number of cusps on a cubic.
  • KM 44: a cubic with 1 conical singularity (A1) and one higher biplanar singularity (A5) 2 straight lines. We call this surface “Schläfli’s cubic” because Schläfli was the first to realize that a cubic may contain an A5 and an A1 singularity, but no higher A-singularity, in particular no A6.

Buy each model individually

To put each of the 45 models from “45 cubics series II (cylinder cut)” invididually into your shapeways cart, you may use the following links:

Below you will find other posts related to cubic surfaces: