On this page, we present our most recent news, ordered by date. For browsing our catalogue of math sculptures in a more structured way, either use the menu bar or a search field.
February 2017
Smoothed Togliatti quintic
A Togliatti quintic surface is a so-called world record surface. Among all quintic surfaces, it has the maximum possible number of singularities, namely 31. Our model is a smoothed version of such a surface.
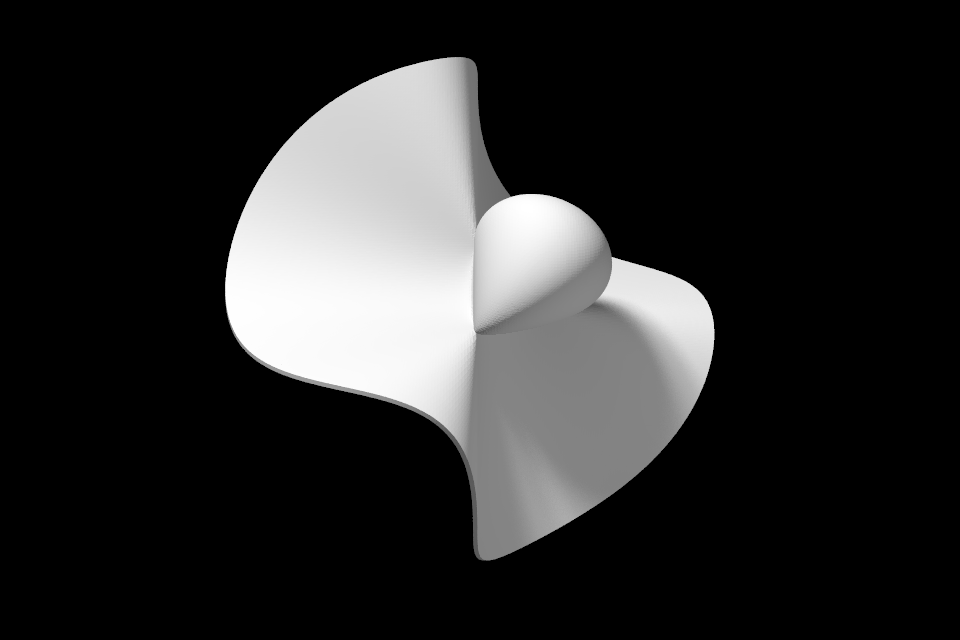
Four pillows meet pendant
The shape of our "four pillows meet pendant" is given by a single mathematical equation. Have you ever seen a pendant like this before?
Six pillows’ secret pendant
Our "six pillows' secret pendant" is a very special piece of math jewelry. Its shape is given by a single equation. Have you ever seen a pendant like this before?
A smoothed Kummer surface
The photo shows a smoothed Kummer surface in steel (inflated with bronze). This post also features links to plastic versions of this shape. The Kummer surface is a classic from the 19th century; our model is a smoothed version of it.
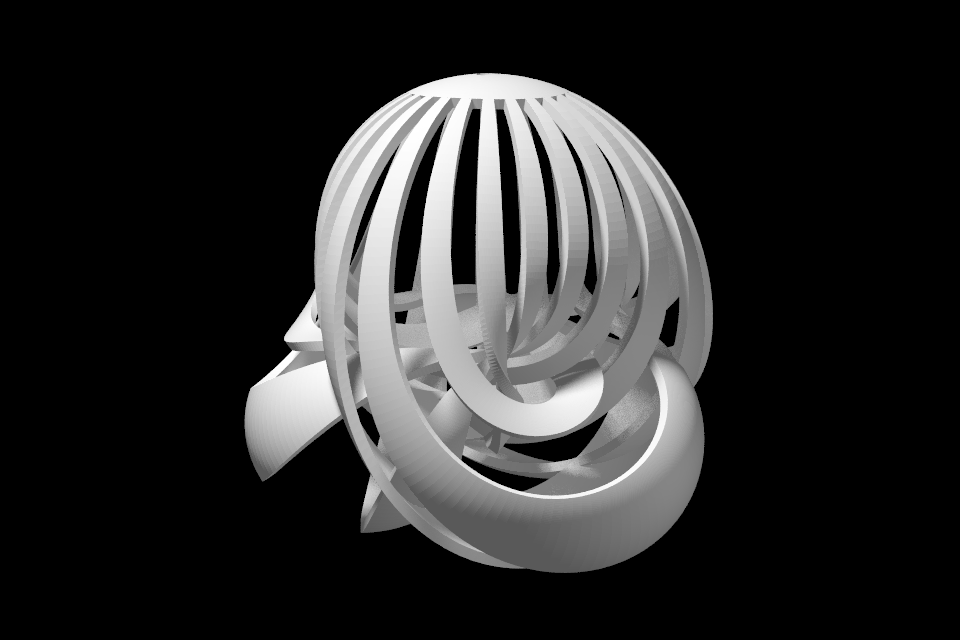
Dancing torus knot
This object looks like a piece of art. But in fact, it is "just" a mathematical curve, called 7/3 torus knot. Imagine a donut, and then tie a cord around it in some interesting way.
Tetrahedral symmetric cubic
Cubic surfaces are classics from the 19th century. This particular cubic is smooth and has tetrahedral symmetry. All 27 lines are real, but only 24 are visible in the model because 3 are infinitly far away.
A boy surface, stripes version
This so-called Boy surface represents a fascinating example of a non-orientable surface. The first such surface was constructed by Werner Boy in his dissertation in 1902.
The Henrici cubic with three cusps
In the 19th century, Olaus Henrici constructed a model of a quite symmetric cubic surface. This is a modern variant of it, allowing also to look "inside" of it from the bottom.
The Clebsch diagonal surface with two planes
This version of Clebsch's famous diagonal surface model features colored lines and two additional planes. One intersects the surfaces in a line and a hyperbola, the another one in three lines.
January 2017
The Cayley/Klein cubic with four singularities in the family room
Our modern version of Klein's historical cubic surface model with four singularities is the main figure in our photo from the series "math sculptures in context". It is the pure white version with its 9 straight lines.
Clebsch and Klein in the family room
It was back in the 1872 Göttingen, Germany, at a meeting of the scientific society. Alfred Clebsch and Felix Klein each presented a model of a cubic surface. Our modern versions of these historical - nowadays quite famous - sculptures are the main figures in our photo.
The Clebsch diagonal surface in the family room
Our modern version of Clebsch's historical - nowadays quite famous - diagonal surface model is the main figure in our photo from the series "math sculptures in context". It is the pure white version with the 27 straight lines.