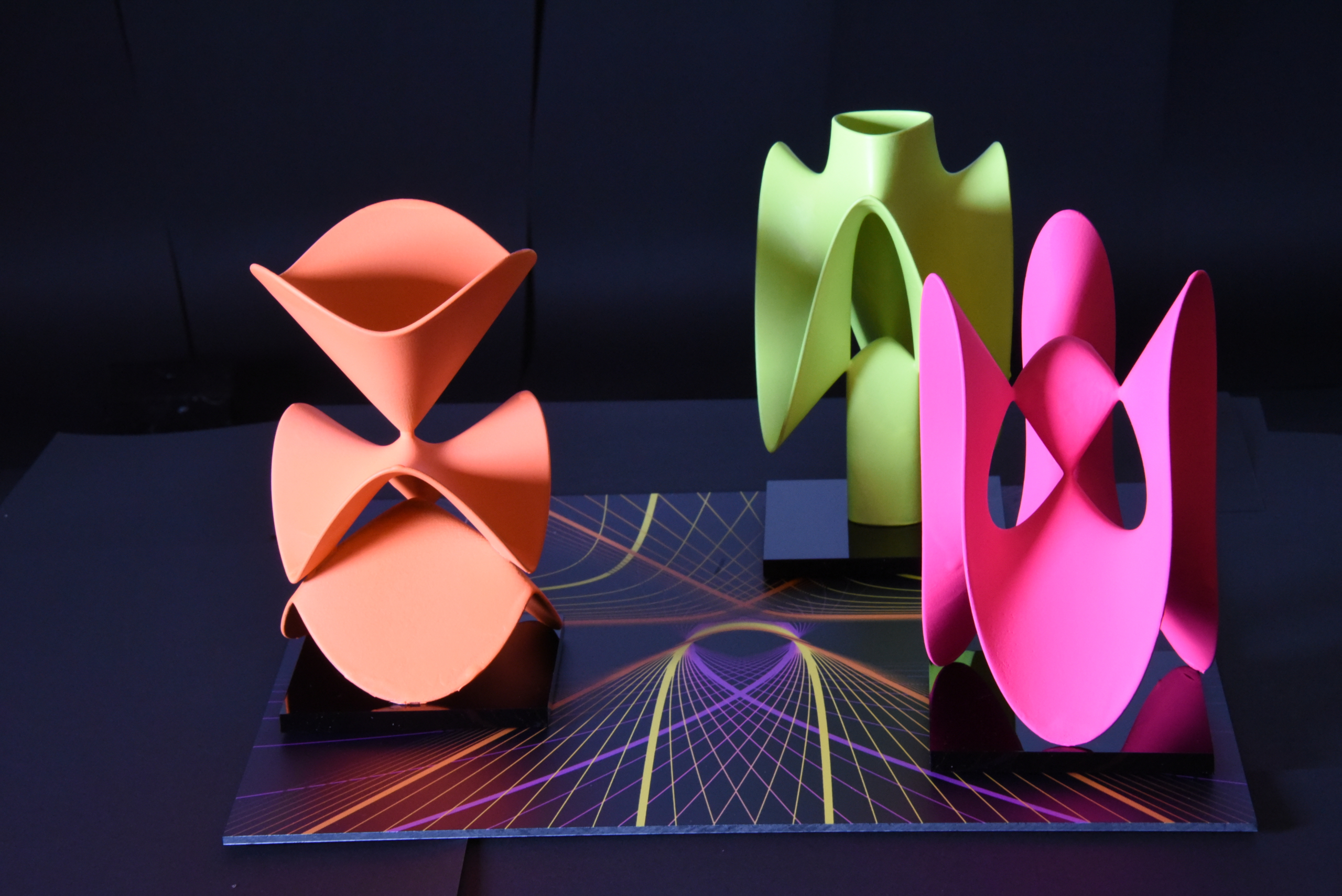
In January 2025, Oliver Labs created a corresponding group object composed of three 3d printed sculptures standing on a planar visualization of this family of curves for an exhibition at the Planetarium, Hamburg, Germany. See their website for a brief intro (in German) to the exhibition (from January 25, 2025 to May 31, 2025), and for the exhibition book as a pdf.
This piece of math art visualizes one of the mathematical ways to work with infinity: The so-called “projective space” is essentially a way to treat all views of an object in 3d in a single piece by considering a related object in four dimensions, here a so-called “projective cubic surface”.
The piece of math art shows three of these infinitely many 3d views of this projective object living in 4d. All three have 4 special points, called “singular points” (the thin points of the sculptures where two conical parts meet in a “point”). With the yellow surface, one of these singular points is at infinity, so only three singular points are visible in this sculpture. With the pink/magenta sculpture, the four singular points connect the central tetrahedral part with the outer parts.
The fact that all three surfaces come from a family of infinitely many related objects is illustrated by the family of planar curves on which the three sculptures have been placed. These curves are vertical plane cuts through the central part of some of the infinitely many surfaces of the family, of which the three thick curves are plane cuts of the three sculptures.
In the following days and weeks, we will write some posts explaining some of the aspects visualized by the piece of math art.
In particular, in these posts, we will explain how the following videos relate to the group object shown above. The brief sentences below are only intended as first ideas on what the videos are about. More details will follow in subsequent posts.
Illustrating Videos
The following video shows a family of affine views of a projective cubic surface.
The following video shows a planar section of a family of affine views of a projective cubic surface.
The following videos show a double cone with its singular point moving towards infinity and back.
This is similar to parallel streets seemingly meeting at the horizon, as illustrated in the second video.




Leave A Comment